Finding Zero (3 page)
Authors: Amir D. Aczel

I learned more about numbers from this lover of mathematics than I ever did at school, and I was grateful to him. But the mystery of where the ten numerals we use today originally came from continued to haunt me. At the same time, as I matured through school and pursued adventures to discover numerals while traveling the Mediterranean aboard my father's ship, I began to understand the abstractâand even more mysteriousâconcept of a number. I realized that 3, for example, stood for the
idea
of “threeness”âsomething that was shared by all things in the universe that were three. All of them were to be described, in their quality of threeness, by the unique symbol 3. Equally, 5 stood for the quality of “fiveness” shared by everything that was five in number. This fascinating discovery made me even more eager to find where the numerals came from since they actually stood for something even deeper and more alluring than I could have ever imagined as a young child. I wanted to dedicate my life to traveling the world in search of an answer to the origin of numbers. Who
invented these wondrous ten numerals? I asked myself this question all the time, and also, Who ever came up with the amazing idea that a concept of “threeness” or “seventeenness” or “three-hundred-and-five-ness” could be captured simply by a combination of ten signs arranged in certain ways?
In 1972, after high school and the obligatory service in the Israeli army, I was accepted as an undergraduate in mathematics at the University of California at Berkeley. I now made one more voyage on my father's ship. By then, all the passenger ships had been sold because Zim Lines had lost too much money due to poor management of the company's cruise division, called Zim Passenger Lines, and my father was now the captain of a small, slow, old cargo ship, the
M.V. Yaffo,
which sailed between the Mediterranean and the Americas. So I hitched a ride aboard my father's ship, embarking in Haifa in late July for the long trip that would ultimately bring me to Berkeley.
A cargo ship is as different from a cruise ship as a truck is from a limousine. Like a working truck, a cargo ship can be dirty and dusty, but its cabins are roomy; with no passengers, there's no need to cram many people into limited cabin space, and the crew is therefore more comfortable. But the flipside is that there is nothing to do: no cocktail parties or bars or ballrooms, and no exciting social gatherings. A voyage on a cargo ship can be lonely.
But Laci was still my father's steward, and I was very fond of him. We often talked about mathematics during this trip.
As an adult, I now understood that the mystery that had held my attention since early childhood was really two mysteries. One was where the first numerals originated: In which part of the world, and when, did people first invent the nine numerals, and zero, that with time evolved into the numbers that rule our world? And the second was a deeper conundrum, which I was now sophisticated enough to discern: How did humans abstract the concept of number? How did the idea of a number originate, and how did it develop and mature through history, to bring us to the digitally dominated society in which we live today?
Laci and I spent many hours together discussing this latter idea as the ship slowly made its way across the ocean. It was a far deeper discussion than I had ever had with him as a child, certainly, and he brought to it his full intellectual abilities as a one-time doctoral student of pure mathematics at the top Russian university, where mathematics has always been one of the most important fields of study. It was a pleasure to sit with Laci on deck chairs discussing mathematical concepts I was only beginning to understand and be fascinated byâbuilding on the earlier ideas he had taught me as a child: numerals, numbers, prime numbers, and the mysterious Fibonacci sequence.
Having finally crossed the Atlantic,
we docked for a few days in Halifax, Nova Scotia, and then continued to New York City; Charleston, South Carolina; and finally Miami, where I disembarked before the ship continued on to the Caribbean and
South America. Before I left ship to fly to San Francisco and start my studies, Laci said one last thing to me in parting: “Remember how when you were little we used to talk about where the numerals came from? Maybe you'll find out. I once read in a science magazine that a French archaeologist may have found something about the numbers in Asia decades agoâsomething important relating to the zero. But I don't remember any of the details.”
Laci's parting words intrigued me, but I had no opportunity to pursue this research further. At Berkeley, I had a full plate of math courses, challenging but often enjoyable, and I had to worry about grades and exams and learning to become a mathematician.
However, through my coursesâmostly mathematics, but also anthropology, sociology, and philosophyâI learned a fair amount also about numbers and their development.
Numbers, as a concept, are much older than we might think. In 1960, a Belgian explorer named Jean de Heinzelin de Braucourt was surveying the region of Ishango, at the border of present-day Uganda and Congo (then the Belgian Congo), when he discovered a strange-looking bone: a baboon's fibula bearing what looked like numerous tally marks. Analysis later concluded that these markings might evidence very early counting. The bone has three sets of identical notches, adding, respectively, to the totals 60, 48, and 60. The markings are grouped in several sets containing 5, 7, 9, 11, or 13 tally marks each. This bone was scientifically dated to about 20,000 years agoâthe Paleolithic eraâwhen humans lived in hunter-gatherer groups. The Ishango bone provides some of the earliest known evidence of a form of
counting by humans who lived in Africa so long ago, and it is now displayed at the Royal Belgian Institute of Natural Sciences in Brussels.

The Ishango bone: a baboon fibula, about 20,000 years old, bearing notches believed to represent early evidence of counting by our species.
What does the Ishango bone represent? It seems that in prehistoric times, early humans roaming the bush in Africa used bones of dead animals as a way of drawing the first
one-to-one correspondence
between the number of animals they were able to hunt and notches they made on a piece of bone. This was not quite counting, but it was close. Anyone could see that a bone that had more such tally marks implied that its owner had hunted more animalsâperhaps without really knowing what the actual
number
was. Of course all this is merely a hypothesis, but it is a likely one.
The Ishango bone is certainly the best early example of pre-counting. But there is some evidence that European humans may also have used some kind of pre-counting: In addition to the Ishango bone, several animal bones with markings that are likely
tally counts have been discovered in Europe and also dated to the Paleolithic.
1
A later piece of evidence for something resembling counting comes from the mysterious Neolithic stone arrangements, believed to be about 6,000 years old, at Carnac on the coast of Brittany in France. Interestingly, the megaliths are found in groups, and the groups are often comprised of a prime number of megaliths: 7, 11, 13, and 17. Was this by chance, or does it represent a form of counting? Does it, perhaps, represent an even deeper understanding of numbers? We don't know. Carnac is a mystery that archaeology has never been able to explain, despite attempts over many decades. Nobody knows why so many very heavy stones were placed in rows. Perhaps a connection exists with Stonehenge, where similar stones were placed in circles around the same time.
But Ishango and Carnac do not present what we consider numbers. In everyday life, numbers began with simple representations of a quantity by people using their fingers. Aristotle wrote two-and-a-half millennia ago, “Or is it because men were born with ten fingers and so, because they possess the equivalent of pebbles to the number of their fingers, come to use this number for counting everything else as well?”
2
And since we also have ten toes, early societies used these as well to count beyond ten. Remnants of such a base-20 number system that existed long ago can still be seen in French, through words such as
quatre-vingt
(four twenties) for 80.
Clearly, the form of counting we use evolved from an accident of nature: our having five fingers on each hand and five toes on each foot. Indeed, in some languages, such as Old Khmer, five was used as a point to anchor the other numbers: After four comes five,
then five-and-one, five-and-two, and so on to ten, which becomes the next anchor. When we look at the early European numbers, the Roman numerals, we see the same trend: The Roman numbers IV, V, VI, VII, VIII, are all anchored at five (V), and only after eight do we come to measure them in relation to ten: IX, X, XI, XII, XIII. So the use of five and ten as key numbers evolved in different parts of the world.
In ancient India, the number 10 was the anchor. Decimal numbers were evident very early on there, as early as the sixth century BCE as observed in some inscriptions. And once they learned the powers of tenâsimply by using ten fingers, and then using ten fingers ten times, as in ten people, one for each finger, each holding up his or her ten fingersâthe Indians of antiquity understood that this process can go on forever. Ten times ten people holding their fingers up was ten to the third power, and ten times ten times ten people with ten fingers each was ten to the fourth power, and so on without a limit.
During the Han Dynasty in China (206 BCE to 220 CE), a mathematical work titled
Nine Chapters on the Mathematical Art
appeared, employing both positive numbers, colored red, and negative ones, colored black. And in Egypt of the third century, a leading Greek mathematician named Diophantus obtained negative answers to some of his equations but immediately dismissed them as unrealistic. So the idea for negative numbers is quite old, but people did not understand such numbers. The double-entry bookkeeping system used in accounting today was developed in Europe in the thirteenth century in part to avoid using negative numbers. To define negative numbers requires the concept of
zero.
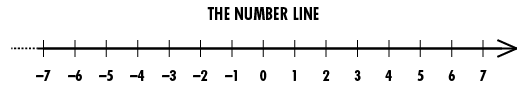
Negative numbers are, in a sense, a reflection across zero of the positive numbers. You can see this if you draw the number line, starting at zero and going to the right to 1, 2, 3, and so on; and then extending the numbers to the left of zero to â1, â2, â3, and onward. The number â1 is a reflection of the number +1 through a mirror placed at zero. But the zero plays many other important roles in mathematics and its applications. And some crucial equations in physics, biology, engineering, economics, and other fields use zero as the key element (Maxwell's equations in physics are a good example).
The ancient Babylonians developed a cumbersome number system based on 60 rather than 10, and without a real zero. In this system, ambiguities arose because of the lack of a zero, and they could only be resolved from the context. As a modern example, if someone told you that something costs six-ninety-five, you would understand it to be $6.95 if you were buying a magazine and $695 if you were purchasing an airplane ticket. With their cumbersome number system, the Babylonians were forced to make such assumptions all the time. But interestingly, vestiges of their ancient system still exist today: We have 60 seconds in a minute, 60 minutes in an hour, and the circle has 360 (6 Ã 60) degrees. All in all,
however, the Babylonian system would be as inadequate today as doing calculations with one's fingers and toes.
An interesting question arises: Why use a base of 60? We have ten fingers, so base 10 makes sense, and if you insist on also counting toes, a base 20 may be useful. But 60? In 1927, the prominent Austrian American historian of science Otto Neugebauer suggested that the choice of the large base of 60 was made to address an important practical problem in using numbers in Babylonia. Often, fractions of a whole, such as
1
â
2
,
1
â
3
,
3
â
4
, and
2
â
3
, were required as measures: Perhaps someone wanted to buy half a loaf of bread, or a third of a wheel of cheese, or two-thirds of a shepherd's pie. How could the numbers
1
â
2
,
1
â
3
,
3
â
4
, and
2
â
3
âthe most commonly used fractionsâbe reconciled with a natural system using ten numbers abstracted from fingers? Neugebauer's answer was that 60 is a good solution since this number is divisible by 2, 3, 4, and 10, and for this reason it was chosen as the base for the entire system. Another hypothesis is that the Babylonians knew five planets (Mercury, Venus, Mars, Saturn, and Jupiter) and that they chose their base, for cosmological reasons, to be the product of this number and the 12 (lunar) months of the year.
3
I already knew something about the Greco-Roman number system from visiting Greece and Rome. This system, too, lacked a zero, and with it the ability of the numbers to cycle so that the same signs could be used over and over again to mean different things. The Greco-Roman system, like the Babylonian and Egyptian, had to fade away, remaining only as an elegant way of commemorating official dates or representing time on clock and watch faces.