Authors: James Gleick
Tags: #Biography & Autobiography, #Science & Technology
Isaac Newton (6 page)
With symbols came equations: relations between quantities, and changeable relations at that. This was new territory, and Descartes exploited it. He treated one unknown as a spatial dimension, a line; two unknowns thus define a plane. Line segments could now be added and even multiplied. Equations generated curves; curves embodied equations. Descartes opened the cage doors, freeing strange new bestiaries of curves, far more varied than the elegant conic sections studied by the Greeks. Newton immediately began expanding the possibilities, adding dimensions, generalizing, mapping one plane to another with new coordinates. He taught himself to find real and complex roots of equations and to factor expressions of many terms—polynomials. When the infinite number of points in a curve correspond to the infinite solutions of its equation, then all the solutions can be seen at once, as a unity. Then equations have not just solutions but other properties: maxima and minima, tangents and areas. These were visualized, and they were named.
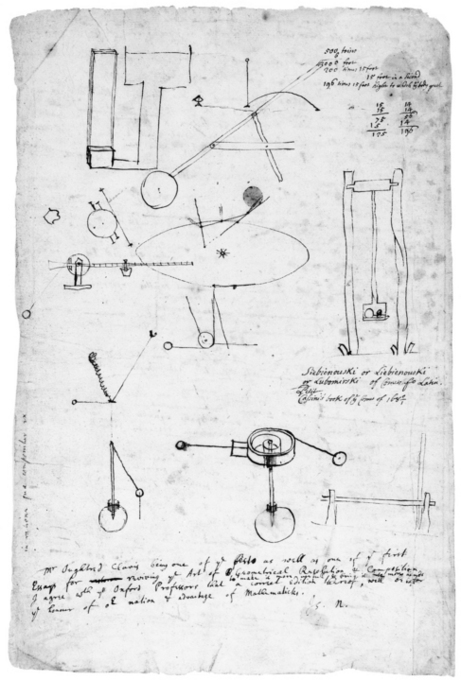
Early Newton drawings of apparatus
.
(illustration credit 3.1)
No one understands the mental faculty we call mathematical intuition; much less, genius. People’s brains do not differ much, from one to the next, but numerical facility seems rarer, more special, than other talents. It has a threshold quality. In no other intellectual realm does the genius find so much common ground with the idiot savant. A mind turning inward from the world can see numbers as lustrous creatures; can find order in them, and magic; can know numbers as if personally. A mathematician, too, is a polyglot. A powerful source of creativity is a facility in translating, seeing how the same thing can be said in seemingly different ways. If one formulation doesn’t work, try another.
Newton’s patience was limitless. Truth, he said much later, was “the offspring of silence and meditation.”
15
And he said: “I keep the subject constantly before me and wait ’till the first dawnings open slowly, by little and little, into a full and clear light.”
16
Newton’s Waste Book filled day by day with new research
in this most abstract of realms. He computed obsessively. He worked out a way to transform equations from one set of axes to any alternative frame of reference. On one page he drew a hyperbola and set about calculating the area under it—“squaring” it. He stepped past the algebra Descartes knew. He would not confine himself to expressions of a few (or many) terms; instead he constructed infinite series: expressions that continue forever.
17
An infinite series need not sum to infinity; rather, because the terms could grow smaller and smaller, they could close in on a goal or limit. He conceived such a series to square the hyperbola—
—and carried out the calculation to fifty-five decimal places: in all, more than two thousand tiny digits marching down a single page in orderly formation.
18
To conceive of infinite series and then learn to manipulate them was to transform the state of mathematics. Newton seemed now to
possess a limitless ability to generalize, to move from one or a few particular known cases to the universe of all cases. Mathematicians had a glimmering notion of how to raise the sum of two quantities,
a
+
b
, to some power. Through infinite series, Newton discovered in the winter of 1664 how to expand such sums to any power, integer or not: the general binomial expansion.
(illustration credit 4.1)
He relished the infinite, as Descartes had not. “We should never enter into arguments about the infinite,” Descartes had written.
For since we are finite, it would be absurd for us to determine anything concerning the infinite; for this would be to attempt to limit it and grasp it. So we shall not bother to reply to those who ask if half an infinite line would itself be infinite, or whether an infinite number is odd or even, and so on. It seems that nobody has any business to think about such matters unless he regards his own mind as infinite.
19
Yet it turns out that the human mind, though bounded in a nutshell, can discern the infinite and take its measure.
A special aspect of infinity troubled Newton; he returned to it again and again, turning it over, restating it with new definitions and symbols. It was the problem of the infinitesimal—the quantity, impossible and fantastic, smaller than any finite quantity, yet not so small as zero. The infinitesimal was anathema to Euclid and Aristotle. Nor was Newton altogether at ease with it.
20
First he thought in terms of “indivisibles”—points which, when added to one another infinitely, could perhaps make up a finite length.
21
This caused paradoxes of dividing by zero:
—nonsensical results if 0 is truly zero, but necessary if 0 represents some indefinitely small, “indivisible” quantity. Later he added an afterthought—
(that is undetermined)
Tis indefinitehow greate a sphære may be made how greate a number may be reckoned, how far matter is divisible, how much time or extension wee can fansy but all the Extension that is, Eternity,
are infinite.
22
—blurring the words
indefinite
and
undetermined
by applying them alternately to mathematical quantities and degrees of knowledge. Descartes’s reservations notwithstanding, the infinitude of the universe was in play—the boundlessness of God’s space and time. The infinitesimal—the almost nothing—was another matter. It might have been simply the inverse problem: the infinitely large and the infinitely small. A star of finite size, if it could be seen at an infinite distance, would appear infinitesimal. The terms in Newton’s infinite series approached the infinitesimal. “We are among infinities and indivisibles,” Galileo said, “the former incomprehensible to our understanding by reason of their largeness, and the latter by their smallness.”
23
Newton was seeking better methods—more general—for finding the slope of a curve at any particular point, as well another quantity, related but once removed, the degree of curvature, rate of bending, “the crookedness in lines.”
24
He applied himself to the tangent, the straight line that grazes
the curve at any point, the straight line that the curve would
become
at that point, if it could be seen through an infinitely powerful microscope. He drew intricate constructions, more complex and more free than anything in Euclid or Descartes. Again and again he confronted the specter of the infinitesimal: “Then (if
hs
&
cd
have an infinitely little distance otherwise not) …”; “… (which operacon cannot in this case bee understood to bee good unlesse infinite littleness may bee considered geometrically).…”
25
He could not escape it, so he pressed it into service, employing a private symbol—a little o—for this quantity that was and was not zero. In some of his diagrams, two lengths differed “but infinitely little,” while two other lengths had “no difference at all.” It was essential to preserve this uncanny distinction. It enabled him to find areas by infinitely partitioning curves and infinitely adding the partitions. He created “a Method whereby to square those crooked lines which may bee squared”
26
—to
integrate
(in the later language of the calculus).
As algebra melded with geometry, so did a physical counterpart, the problem of motion. Whatever else a curve was, it naturally represented the path of a moving point. The tangent represented the instantaneous direction of motion. An area could be generated by a line sweeping across the plane. To think that way was to think kinetically. It was here that the infinitesimal took hold. Motion was smooth, continuous, unbroken—how could it be otherwise? Matter might reduce to indivisible atoms, but to describe motion, mathematical points seemed more appropriate. A body on its way from
a
to
b
must surely pass through every point between. There must
be
points between,
no matter how close
a
is to b; just as between any pair of numbers, more numbers must be found. But this continuum evoked another form of paradox, as Greek philosophers had seen two thousand years before: the paradox of Achilles and the tortoise. The tortoise has a head start. Achilles can run faster but can never catch up, because each time he reaches the tortoise’s last position, the tortoise has managed to crawl a bit farther ahead. By this logic Zeno proved that no moving body could ever reach any given place—that motion itself did not exist. Only by embracing the infinite and the infinitesimal, together, could these paradoxes be banished. A philosopher had to find the sum of infinitely many, increasingly small intervals. Newton wrestled with this as a problem of words: swifter, slower; least distance, least progression; instant, interval.