100 Essential Things You Didn't Know You Didn't Know (4 page)
Read 100 Essential Things You Didn't Know You Didn't Know Online
Authors: John D. Barrow

fn1
The basket ball rebounds from the ground with speed V and hits the ping-pong ball when it is still falling at speed V. So, relative to the basket ball, the ping-pong ball rebounds upwards at speed 2V after its velocity gets reversed by the collision. Since the basket ball is moving at speed V relative to the ground this means that the ping-pong ball is moving upwards at 2V + V = 3V relative to the ground after the collision. Since the height reached is proportional to V
2
this means that it will rise 3
2
= 9 times higher than in the absence of its collision with the basket ball. In practice, the loss of energy incurred at the bounces will ensure that it rises a little less than this.
10
It’s a Small World After All
It’s a small world but we all run in big circles.
Sasha Azevedo
How many people do you know? Let’s take a round number, like 100, as a good average guess. If your 100 acquaintances each know another 100 different people then you are connected by one step to 10,000 people: you are actually better connected than you thought. After n of these steps you are connected to 10
2(n+1)
people. As of this month the population of the world is estimated to be 6.65 billion, which is 10
9.8
, and so when 2(n+1) is bigger than 9.8 you have more connections than the whole population of the world. This happens when n is bigger than 3.9, so just 4 steps would do it.
This is a rather remarkable conclusion. It makes a lot of simple assumptions that are not quite true, like the one about all your friend’s friends being different from each other. But if you do the counting more carefully to take that into account, it doesn’t make much difference. Just six steps is enough to put you in contact with just about anyone on Earth. Try it – you will be surprised how often fewer than 6 steps links you to famous people.
There is a hidden assumption in this that is not well tested by thinking about your links to the Prime Minister, David Beckham or the Pope. You will be surprisingly close to these famous people because they have many links with others. But try linking to an
Amazonian
Indian tribe member or a Mongolian herdsman and you will find that the chain of links is much longer. You might not even be able to close it. These individuals live in ‘cliques’, which have very simple links once you look beyond their immediate close-knit communities.
If your network of connections is a chain or a circle, then you are only connected by one person on either side and overall connectedness is poor. However, if you are in a ring of these connections with some other random links added, then you can get from one point on the ring to any other very quickly.
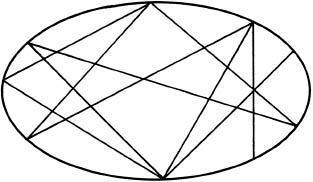
In recent years we have begun to appreciate how dramatic the effects of a few long-range connections can be for the overall connectivity. A group of hubs that produce lots of connections to nearby places can be linked up very effectively by just adding a few long-range connections between them.
These insights are important when it comes to figuring out how much coverage you need in order for mobile phone links between
all
users to be possible, or how a few infected individuals can spread a disease by their interactions with members of a population. When airlines try to plan how they arrange hubs and routes so as to minimise journey times, or maximise the number of cities they can connect with a single change of planes or minimise costs, they need to understand the unexpected properties of these ‘small world’ networks.
The study of connectedness shows us that the ‘world’ exists at many levels: transport links, phone lines, email paths all create networks of interconnections that bind us together in unlikely ways. Everything is rather closer than we thought.
11
Bridging That Gap
Like a bridge over troubled water.
Paul Simon and Art Garfunkel
One of the greatest human engineering achievements has been the construction of bridges to span rivers and gorges that would otherwise be impassable. These vast construction projects often have an aesthetic quality about them that places them in the first rank of modern wonders of the world. The elegant Golden Gate Bridge, Brunel’s remarkable Clifton Suspension Bridge and the Ponte Hercilio Luz in Brazil have spectacular shapes that look smooth and similar. What are they?
There are two interesting shapes that appear when weights and chains are suspended and they are often confused or simply assumed to be the same. The oldest of these problems was that of describing the shape that is taken up by a hanging chain or rope whose ends are fixed at two points on the same horizontal level. You can see the shape easily for yourself. The first person to claim they knew what this shape would be was Galileo, who in 1638 maintained that a chain hanging like this under gravity would take up the shape of a parabola (this has the graph y
2
= Ax where A is any positive number). But in 1669 Joachim Jungius, a German mathematician who had special interests in the applications of mathematics to physical problems, showed him to be wrong. The determination of the actual equation for the hanging
chain
was finally calculated by Gottfried Leibniz, Christiaan Huygens, David Gregory and Johann Bernoulli in 1691 after the problem had been publicly announced as a challenge by Johann Bernoulli a year earlier. The curve was first called the
catenaria
by Huygens in a letter to Leibniz, and it was derived from the Latin word
catena
for ‘chain’, but the introduction of the anglicised equivalent ‘catenary’ seems to have been due to the US President Thomas Jefferson in a letter to Thomas Paine, dated 15 September 1788, about the design of a bridge. Sometimes the shape was also known as the
chainette
or
funicular
curve.
The shape of a catenary reflects the fact that its tension supports the weight of the chain itself and the total weight born at any point is therefore proportional to the total length of chain between that point and the lowest point of the chain. The equation for the hanging chain has the form y = Bcosh(x/B) where B is the constant tension of the chain divided by its weight per unit length.
1
If you hold two ends of a piece of hanging chain and move them towards each other, or apart, then the shape of the string will continue to be described by this formula but with a different value of B for each position. This curve can also be derived by asking for the shape that makes the centre of gravity of the suspended chain as low as possible.
Another spectacular example of a man-made catenary can be seen in St Louis, Missouri, where the Gateway Arch is an upside-down catenary (
see here
). This is the optimal shape for a self-supporting arch, which minimises the shear stresses because the stress is always directed along the line of the arch towards the ground. Its exact mathematical formula is written inside the arch. For these reasons, catenary arches are often used by architects to optimise the strength and stability of structures; a notable example is in the soaring high arches of Antoni Gaudí’s unfinished Sagrada Familia Church in Barcelona.
Another beautiful example is provided by the Rotunda building designed by John Nash in 1819 to be the Museum of Artillery,
located
on the edge of Woolwich Common in London. Its distinctive tent-like roof, influenced by the shape of soldiers’ bell tents, has the shape of one half of a catenary curve.

John Nash’s Rotunda building
There is, however, a big difference between a hanging chain and a suspension bridge like the Clifton or the Golden Gate. Suspension bridges don’t only have to support the weight of their own cables or chains. The vast bulk of the weight to be supported by the bridge cable is the deck of the bridge. If the deck is horizontal with a constant density and cross-sectional area all the way along it, then the equation for the shape of the supporting cable is now a parabola y = x
2
/2B, where B is (as for the hanging chain equation) a constant equal to the tension divided by the weight per unit length of the bridge deck.
One of the most remarkable is the Clifton Suspension Bridge in Bristol, designed by Isambard Kingdom Brunel in 1829 but completed only in 1865, three years after his death. Its beautiful parabolic form remains a fitting monument to the greatest engineer since Archimedes.

The St Louis Gateway Arch
12
On the Cards
Why don’t children collect things anymore? Whatever happened to those meticulously kept stamp albums . . . ?
Woman’s Hour
, BBC Radio 4
Last weekend, hidden between books in the back of my bookcase, I came across two sets of cards that I had collected as a young child. Each set contained fifty high-quality colour pictures of classic motor cars with a rather detailed description of their design and mechanical specification on the reverse. Collecting sets of cards was once all the rage. There were collections of wartime aircraft, animals, flowers, ships and sportsmen – since these collections all seemed to be aimed at boys – to be amassed from buying lots of packets of bubble gum, breakfast cereals or packets of tea. Of the sports cards, just as with today’s Panini ‘stickers’, the favoured game was football (in the US it was baseball), and I always had my suspicions about the assumption that all the players’ cards were produced in equal numbers. Somehow everyone seemed to be trying to get the final ‘Bobby Charlton’ card that was needed to complete the set. All the other cards could be acquired by swopping duplicates with your friends, but everyone lacked this essential one.
It was a relief to discover that even my own children engaged in similar acquisitive practices. The things collected might change but the basic idea was the same. So what has mathematics got to
do
with it? The interesting question is to ask how many cards we should expect to have to buy in order to complete the set, if we assume that each of them is produced in equal numbers and so has an equal chance of being found in the next packet that you open. The motor car sets I came across each contained 50 cards. The first card I get will always be one I haven’t got but what about the second card? There is a 49/50 chance that I haven’t already got it. Next time it will be a 48/50 chance and so on.