Hyperspace (16 page)

When Einstein was 26 years old, he calculated precisely how energy must change if the relativity principle was correct, and he discovered the relation
E
=
mc
²
. Since the speed of light squared (
c
²
) is an astronomically large number, a small amount of matter can release a vast amount of energy. Locked within the smallest particles of matter is a storehouse of energy, more than 1 million times the energy released in a chemical
explosion. Matter, in some sense, can be seen as an almost inexhaustible storehouse of energy; that is, matter is condensed energy.

In this respect, we see the profound difference between the work of the mathematician (Charles Hinton) and that of the physicist (Albert Einstein). Hinton spent most of his adult years trying to visualize higher
spatial
dimensions. He had no interest in finding a physical interpretation for the fourth dimension. Einstein saw, however, that the fourth dimension can be taken as a
temporal
one. He was guided by a conviction and physical intuition that higher dimensions have a purpose: to unify the principles of nature. By adding higher dimensions, he could unite physical concepts that, in a three-dimensional world, have no connection, such as matter and energy.

From then on, the concept of matter and energy would be taken as a single unit: matter-energy. The direct impact of Einstein’s work on the fourth dimension was, of course, the hydrogen bomb, which has proved to be the most powerful creation of twentieth-century science.

Einstein, however, wasn’t satisfied. His special theory of relativity alone would have guaranteed him a place among the giants of physics. But there was something missing.

Einstein’s key insight was to use the fourth dimension to unite the laws of nature by introducing two new concepts: space-time and matter-energy. Although he had unlocked some of the deepest secrets of nature, he realized there were several gaping holes in his theory. What was the relationship between these two new concepts? More specifically, what about accelerations, which are ignored in special relativity? And what about gravitation?

His friend Max Planck, the founder of the quantum theory, advised the young Einstein that the problem of gravitation was too difficult. Planck told him that he was too ambitious: “As an older friend I must advise you against it for in the first place you will not succeed; and even if you succeed, no one will believe you.”
⁵
Einstein, however, plunged ahead to unravel the mystery of gravitation. Once again, the key to his momentous discovery was to ask questions that only children ask.

When children ride in an elevator, they sometimes nervously ask, “What happens if the rope breaks?” The answer is that you become weightless and float inside the elevator, as though in outer space, because both you and the elevator are falling at the same rate. Even
though both you and the elevator are accelerating in the earth’s gravitational field, the acceleration is the same for both, and hence it appears that you are weightless in the elevator (at least until you reach the bottom of the shaft).

In 1907, Einstein realized that a person floating in the elevator might think that someone had mysteriously turned off gravity. Einstein once recalled, “I was sitting in a chair in the patent office at Bern when all of a sudden a thought occurred to me: ‘If a person falls freely he will not feel his own weight.’ I was startled. This simple thought made a deep impression on me. It impelled me toward a theory of gravitation.”
⁶
Einstein would call it “the happiest thought of my life.”

Reversing the situation, he knew that someone in an accelerating rocket will feel a force pushing him into his seat, as though there were a gravitational pull on him. (In fact, the force of acceleration felt by our astronauts is routinely measured in
g’s
—that is, multiples of the force of the earth’s gravitation.) The conclusion he reached was that someone accelerating in a speeding rocket may think that these forces were caused by gravity.

From this children’s question, Einstein grasped the fundamental nature of gravitation:
The laws of nature in an accelerating frame are equivalent to the laws in a gravitational field
. This simple statement, called the
equivalence principle
, may not mean much to the average person, but once again, in the hands of Einstein, it became the foundation of a theory of the cosmos.

(The equivalence principle also gives simple answers to complex physics questions. For example, if we are holding a helium balloon while riding in a car, and the car suddenly swerves to the left, our bodies will be jolted to the right, but which way will the balloon move? Common sense tells us that the balloon, like our bodies, will move to the right. However, the correct resolution of this subtle question has stumped even experienced physicists. The answer is to use the equivalence principle. Imagine a gravitational field pulling on the car from the right. Gravity will make us lurch us to the right, so the helium balloon, which is lighter than air and always floats “up,” opposite the pull of gravity, must float to the left, into the direction of the swerve, defying common sense.)

Einstein exploited the equivalence principle to solve the long-standing problem of whether a light beam is affected by gravity. Ordinarily, this is a highly nontrivial question. Through the equivalence principle, however, the answer becomes obvious. If we shine a flashlight inside an accelerating rocket, the light beam will bend downward toward the floor (because the rocket has accelerated beneath the light beam during the
time it takes for the light beam to move across the room). Therefore, argued Einstein, a gravitational field will also bend the path of light.

Einstein knew that a fundamental principle of physics is that a light beam will take the path requiring the least amount of time between two points. (This is called Fermat’s least-time principle.) Ordinarily, the path with the smallest time between two points is a straight line, so light beams are straight. (Even when light bends upon entering glass, it still obeys the least-time principle. This is because light slows down in glass, and the path with the least time through a combination of air and glass is now a bent line. This is called refraction, which is the principle behind microscopes and telescopes.)
^*

However, if light takes the path with the least time between two points, and light beams bend under the influence of gravity, then the shortest distance between two points is a curved line. Einstein was shocked by this conclusion: If light could be observed traveling in a curved line, it would mean that
space itself is curved
.

At the core of Einstein’s belief was the idea that “force” could be explained using pure geometry. For example, think of riding on a merry-go-round. Everyone knows that if we change horses on a merry-go-round, we feel a “force” tugging at us as we walk across the platform. Because the outer rim of the merry-go-round moves faster than the center, the outer rim of the merry-go-round must shrink, according to special relativity. However, if the platform of the merry-go-round now has a shrunken rim or circumference, the platform as a whole must be curved. To someone on the platform, light no longer travels in a straight line, as though a “force” were pulling it toward the rim. The usual theorems of geometry no longer hold. Thus the “force” we feel while walking between horses on a merry-go-round can be explained as the curving of space itself.

Einstein independently discovered Riemann’s original program, to give a purely geometric explanation of the concept of “force.” We recall
that Riemann used the analogy of Flatlanders living on a crumpled sheet of paper. To us, it is obvious that Flatlanders moving over a wrinkled surface will be incapable of walking in a straight line. Whichever way they walk, they will experience a “force” that tugs at them from left and right. To Riemann, the bending or warping of space causes the appearance of a force. Thus forces do not really exist; what is actually happening is that space itself is being bent out of shape.

The problem with Riemann’s approach, however, was that he had no idea specifically how gravity or electricity and magnetism caused the warping of space. His approach was purely mathematical, without any concrete physical picture of precisely how the bending of space was accomplished. Here Einstein succeeded where Riemann failed.

Imagine, for example, a rock placed on a stretched bedsheet. Obviously the rock will sink into the sheet, creating a smooth depression. A small marble shot onto the bedsheet will then follow a circular or an elliptical path around the rock. Someone looking from a distance at the marble orbiting around the rock may say that there is an “instantaneous force” emanating from the rock that alters the path of the marble. However, on close inspection it is easy to see what is really happening: The rock has warped the bedsheet, and hence the path of the marble.

By analogy, if the planets orbit around the sun, it is because they are moving in space that has been curved by the presence of the sun. Thus the reason we are standing on the earth, rather than being hurled into the vacuum of outer space, is that the earth is constantly warping the space around us (
Figure 4.1
).

Einstein noticed that the presence of the sun warps the path of light from the distant stars. This simple physical picture therefore gave a way in which the theory could be tested experimentally. First, we measure the position of the stars at night, when the sun is absent. Then, during an eclipse of the sun, we measure the position of the stars, when the sun is present (but doesn’t overwhelm the light from the stars). According to Einstein, the apparent relative position of the stars should change when the sun is present, because the sun’s gravitational field will have bent the path of the light of those stars on its way to the earth. By comparing the photographs of the stars at night and the stars during an eclipse, one should be able to test this theory.

This picture can be summarized by what is called Mach’s principle, the guide Einstein used to create his general theory of relativity. We recall that the warping of the bedsheet was determined by the presence of the rock. Einstein summarized this analogy by stating: The presence of matter-energy determines the curvature of the space-time surrounding it. This is the essence of the physical principle that Riemann failed to discover, that the bending of space is directly related to the amount of energy and matter contained within that space.

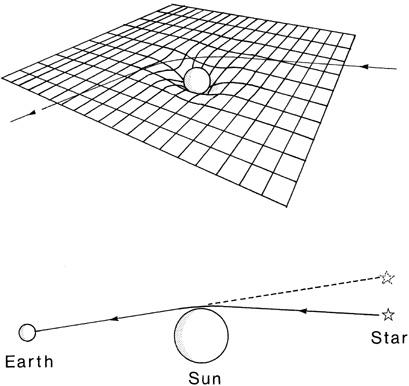
Figure 4.1. To Einstein, “gravity” was an illusion caused by the bending of space. He predicted that starlight moving around the sun would be bent, and hence the relative positions of the stars should appear distored in the presence of the sun. This has been verified by repeated experiments
.

This, in turn, can be summarized by Einstein’s famous equation,
⁷
which essentially states:

Matter-energy → curvature of space-time

where the arrow means “determines.” This deceptively short equation is one of the greatest triumphs of the human mind. From it emerge the principles behind the motions of stars and galaxies, black holes, the Big Bang, and perhaps the fate of the universe itself.

Nevertheless, Einstein was still missing a piece of the puzzle. He had discovered the correct physical principle, but lacked a rigorous mathematical formalism powerful enough to express this principle. He lacked a version of Faraday’s fields for gravity. Ironically, Riemann had the mathematical apparatus, but not the guiding physical principle. Einstein, by contrast, discovered the physical principle, but lacked the mathematical apparatus.

Because Einstein formulated this physical principle without knowing of Riemann, he did not have the mathematical language or skill with which to express his principle. He spent 3 long, frustrating years, from 1912 to 1915, in a desperate search for a mathematical formalism powerful enough to express the principle. Einstein wrote a desperate letter to his close friend, mathematician Marcel Grossman, pleading, “Grossman, you must help me or else I’ll go crazy!”
⁸

Fortunately, Grossman, when combing through the library for clues to Einstein’s problem, accidentally stumbled on the work of Riemann. Grossman showed Einstein the work of Riemann and his metric tensor, which had been ignored by physicists for 60 years. Einstein would later recall that Grossman “checked through the literature and soon discovered that the mathematical problem had already been solved by Riemann, Ricci, and Levi-Civita…. Riemann’s achievement was the greatest one.”

To his shock, Einstein found Riemann’s celebrated 1854 lecture to be the key to the problem. He found that he could incorporate the entire body of Riemann’s work in the reformulation of his principle. Almost line for line, the great work of Riemann found its true home in Einstein’s principle. This was Einstein’s proudest piece of work, even more than his celebrated equation
E
=
mc
²
. The physical reinterpretation of Riemann’s famous 1854 lecture is now called
general relativity
, and Einstein’s field equations rank among the most profound ideas in scientific history.