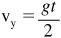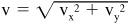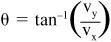125 Physics Projects for the Evil Genius (10 page)

If this section contains more math than you care to do, fast forward directly to the tables in the next section.

Figure 10-1
shows a punted football from the eyes of a physicist.

1. Find the horizontal velocity (in m/s), v
_x
, by dividing the overall distance,
R
, by the total time (hang time, t).

v
_x
= R/t

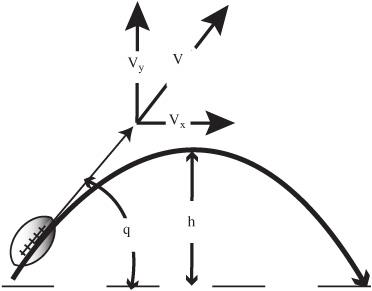
Figure 10-1
Distance, height, velocity, and angle for a football
.

2. Find the vertical velocity (in m/s) by multiplying one half of the hang time (or the time to reach the peak) by the gravitational constant:

where
g
is 9.8m/s
²
.

3. Find the velocity (in m/s) using:

4. Find the angle using:

(In case you don’t know what tan
⁻¹
is you can just use the key on your calculator with that identification. The function, tan
⁻¹
, also called the arctan, gives the angle if you have the
tangent
of that
angle
. You can get the tangent by dividing v
_y
by v
_x
.)

Find (or look up) the velocity, height reached, and angle launched.

See
Tables 10-1
to
10-3
.

Table 10-1
How fast it goes (in m/s)

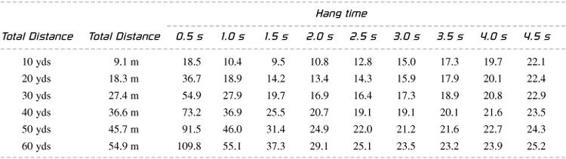
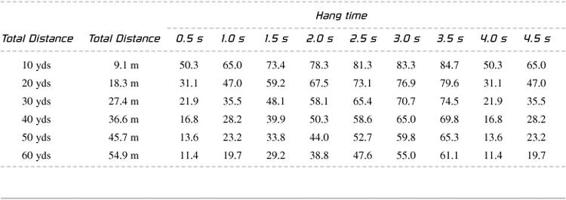
Table 10-2
How high it gets (in m)

Table 10-3
What angle it goes off at (in degrees). Calculations are based on θ = tan
⁻¹
(v
_y
/v
_x
)

This works for the same reasons as the previous experiment. Because horizontal and vertical motion are independent, the range and time in the air can uniquely be determined by the velocity, height, and launch angle.

Determine the velocity, maximum height, and angle for the following cases:

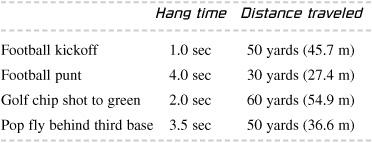
The results are shown in the following table:

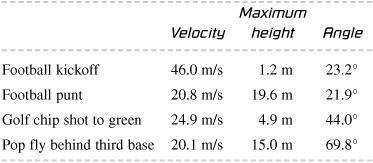
Knowing only the time a projectile is in the air and the distance along the ground that it travels, it is possible to determine the velocity, maximum height, and angle of the projectile.

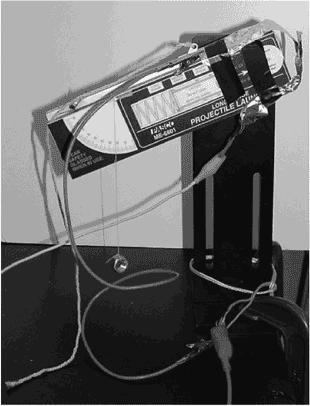
A monkey is hanging from a branch in a tree. The monkey looks hungry and you want to throw a coconut to him. However, the monkey is nervous and, as soon as he sees something being thrown at him, he lets go of the branch. (The monkey apparently knows that in previous versions of this problem, a hunter was trying to shoot it, so the monkey is understandably a bit nervous.) Knowing the monkey will let go as soon as the coconut is thrown, where should you aim? a) Above the monkey b) At the monkey c) Below the monkey.

• “monkey”—(represented by a pie pan or lid of a metal container). See
  Figure 11-1
  .
  
• “coconut”—(represented by a projectile from
  Project 8
  )
  
• DC power supply
  
• electromagnet
  
• insulated wire—about 25 feet
  
• switch that opens the circuit at the precise moment the projectile is launched. This can be accomplished by assembling two pieces of metal foil in front of the launcher. At the instant the projectile emerges, it pushes the foil apart, opening the circuit. See
  Figure 11-2
  for a simple way to set this up. There are also optical techniques to do this, some of which are commercially available.
  

Figure 11-1
The “monkey”: a metal lid held by an electromagnet attached to a vertical piece of wood
.