125 Physics Projects for the Evil Genius (33 page)
Read 125 Physics Projects for the Evil Genius Online
Authors: Jerry Silver

Inelastic collision. Sticking together
.
When objects collide, they either bounce off each other or they stick together.
- 2 low-friction carts
- Velcro or duct tape
- low-friction track (optional)
- motion sensor
- index card
- tape
- Measure the mass of each of the two carts.
- Attach Velcro to the end of each of the carts, so when they meet, they stick together. You could also use duct tape formed into a loop and attached sticky-side out to each of the carts.
- Set up the motion sensor at one end of the table.
- Place the first cart near the motion sensor. The Velcro side should be in front, away from the motion sensor. If you have a low-friction track, place the cart on the track in a line pointing away from the motion sensor.
- Place the second cart near the midpoint of the table with the Velcro in the rear.
- It may be helpful to attach an index card to the back of the first cart to make it easier for the motion sensor to pick it up. If you can get away without doing this, you can avoid air resistance that could slightly affect your result.
- Set up the motion sensor to read distance and velocity versus time.
- Start the motion sensor. It should be on the cart setting and focused on the card of the first cart.
- Give the first cart a push in the direction of the second cart. It should be slow enough to get a good reading from the motion sensor, but fast enough to rear-end the second cart and push it along for at least a few seconds or more. The first cart collides with the second totally
inelastically
, which means they stick together after the collision. - When both carts stop moving, stop collecting data from the motion sensor.
- From the motion-sensor graphs, find the velocity of the first cart before the collision and the velocity of both carts joined together after the collision. The graph of velocity versus time may be a little erratic right after the collision, reflecting the impact. Pick a point where the velocity has settled down.
- Momentum is defined as mass times velocity. Compare the momentum before and after the collision.
- Kinetic energy is defined as ½ times the mass times the velocity squared. Compare the kinetic energy before and the kinetic energy after the collision.

Figure 53-1
Inelastic collision with one or both carts initially moving. Courtesy PASCO
.
The experimental setup is shown in
Figure 53-1
. (This actually shows a motion sensor at
both
ends. The previous procedure uses only one motion sensor, but this can easily extended to include both carts in motion. For simplicity, we will start out with one of the carts stationary.)
The momentum of
both carts before
the collision should equal the momentum of
both carts after
the collision.
Before the collision, one of the carts is stationary, which means it has no momentum, so the moving cart is the only one with momentum before the collision.
After the collision, both carts stick together and move off with the same velocity. The combined mass of the two carts together times their combined velocity is the momentum after the collision.
Figure 53-2
shows the position versus the time graph before and after the collision obtained by a motion sensor. Notice how the slope of the line abruptly drops, indicating the collision.
Figure 53-3
shows the velocity versus time graph before and after the collision. The velocity before and after can be determined directly from the graph. You can notice a slight downward slope indicating some slowing of the carts due to friction. This is not a showstopper for the experiment, but it shows the extent to which an air track can improve the overall results.
The most reliable velocity measurement is immediately before the collision. The collision shows some bouncing around and variability in the velocity for a short period until the two carts stick together and move as one. This provides some insight into the nature of inelastic collisions, which result in the loss of kinetic energy (but not linear momentum). The most reliable postcollision velocity to use is the point where a new horizontal line begins. The results should be fairly accurate, but some losses due to friction may be encountered without an air track. Also, excessive mass can load down the wheel bearing and increase the losses to friction.

Figure 53-2
Motion sensor measurement of distance versus time for an inelastic collision between a moving car and a stationary cart
.
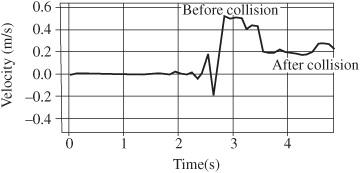
Figure 53-3
Motion sensor measurement of velocity versus time for an inelastic collision between a moving car and a stationary cart
.
The total momentum before an inelastic collision equals the total momentum after.
However, unlike an elastic collision, the kinetic energy for an inelastic collision is less after the collision.
- If you have two motion sensors, you can repeat this with both carts initially in motion. You can get the velocity of each cart right before the carts collide and the velocity of both carts together after the collision. Both sensors will read a positive velocity before the collision as the distance from the sensor increases. After the collision, one sensor reads a positive velocity, while the other reads a negative velocity. Results are shown in
Figure 53-4
. - Compare elastic and inelastic collisions using so-called “happy/sad” balls. The balls appear to be completely identical. However, one is elastic and bounces back from the floor, while the other is inelastic and doesn’t bounce at all.
- Hang elastic and inelastic balls to form a pendulum. Stand up a wooden block in front of the pendulum. Swing the inelastic ball first, so it doesn’t quite knock the block over. Compare that to what happens with the elastic ball swung under the same conditions. Elastic collisions result in
double
the change in momentum as an inelastic collision under the same conditions. This is because in the elastic collision, the momentum not only stops (as it does in the case of an inelastic collision), but it also reverses itself in the other direction.
In an elastic collision, the objects bounce off each other in such as way that linear momentum and kinetic energy are conserved. This is true to the extent that no external force occurs during the collision.
In an inelastic collision, the objects interact in such a way that linear momentum is conserved (as long as no forces affect the collision). However, kinetic energy is not conserved in an inelastic collision. In a perfectly inelastic collision, the objects stick together and move after the collision as if they were a single object.

Figure 53-4
Motion sensor measurement of velocity versus time for an inelastic collision between a moving cart and a second moving cart
.
Impulse and momentum. Eggstreme physics
.
What do you think will happen if you throw a raw egg as hard as you can at a blanket held vertically? There is really only one way to find out. This experiment gives you an opportunity to explore the relationship between momentum and impulse.
- 1 raw egg
- blanket
- 3 people
- mop and paper towels for cleanup (very optional)
1. Hold the blanket vertically, with the bottom edge curled out to form an overhang, as shown in
Figure 54-1
. This requires at least two people.
Figure 54-1
Throwing a raw egg at a blanket
.
Expected Results2. The third person throws the egg at the blanket. Don’t hold back. Give it a good shot. You can throw as hard as you can without having the egg break in your hands as you throw.
You know what will happen if you throw a raw egg against a cinder-block wall. However, if the egg is stopped by the blanket, the deceleration occurs over a sufficiently long time, which prevents the egg from breaking.
Momentum is changed by a force exerted over time. The ability to change an object’s momentum is called
impulse
, which is defined as the force exerted multiplied by the time. Anytime an object is brought to rest, the change in momentum equals the momentum the object had to start, applied in the opposite direction. The impulse to bring that object to rest can come from any combination of force and time, which when multiplied, equal the momentum change.