Chances Are (25 page)
Authors: Michael Kaplan

The Joshua to Galton's Moses was a young man named Karl Pearson. If Francis Galton was the last representative of the great amateur naturalist tradition of gentleman scholars, Pearson was of the rising class of academic professionals: assertive, ambitious, territorial. He had studied in Germany in the 1870s, re-casting his Christian name from Charles, in deference to Marx. He had founded young people's socialist discussion groups, where men and women could converse unchaperoned over tea and buns. His doctoral subject was political scienceâbut he always understood “science” to be what was then current in German universities: the discipline that has since colonized the world, emphasizing laboratory work, uniform method, careful measurement, clear recording of raw data, and application of overt tools of analysis. This science needed ways to relate measurements, not just correct them, as Galileo and Tycho had done. “Correlation,” a word that Galton had invented almost in passing, was the goal: finding the curve that best approximated the relative values of two or more sets of measurements and judging how far off that curve they layâthus determining the degree of confidence a scientist could place in the assumption that they meant something to each other.
Pearson, already a professor of mathematics at University College London, first took on this task with the analysis of an odd distribution of measurements of the foreheads of Neapolitan crabs that troubled his colleague W.F.R. Weldon. Using analytical tools borrowed from mechanics, he was able to resolve this into two superimposed normal distributions, implying that here was a population observed at the moment of evolving into two distinct forms. Sadly, the data may have been at fault, not accounting sufficiently for the crabs' usual habit of snipping bits off one another.
Pearson went on to derive from a single differential equation five Types or families of distribution, both skew and symmetrical, of which the Normal was Type V; where it appeared “we may probably assume something approaching a stable condition; there is production and destruction impartially around the mean.” The young pea plants of exceptional parents will disappoint by their conformism, but the children of the average will also include the exceptionalâlife goes on, churning but stable, down the generations. Other Types governedâor so Pearson confidently claimedâdata “from paupers to cricket-scores”; he fit his curves to St. Louis schoolgirls, barometric pressure, Bavarian skulls, property valuations, divorce, buttercups, and mortality.
You would think that Galton, with his omnivorous delight in the measurable, would have been overjoyed. Here was a man, a “fighting Quaker” like himself, reducing the complexity of the world into forms that might, in time, reveal the inner dynamics of evolution and inheritance. And yet, reading Galton's letters to Pearson, he seems a man who has conjured up a djinn of great power but worrying intent. “[Your charts] are very interesting, but have you got the
rationale
quite clear, for your formulae, and your justification for applying them?” Galton knew he had not the mathematics to dispute Pearson's formulae; but he fretted that a curve expressed as
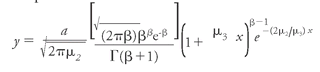 might be difficult to reconcile with the reality of a group of Ovambo warriors, watchful but willing, lining up in their quartiles. Weldon (the man with the crabs) was worried too: “The position now seems to be that Pearson, whom I do not trust as a clear thinker when he writes without symbols, has to be trusted implicitly when he hides in a table of Î-functions, whatever they may be.”
might be difficult to reconcile with the reality of a group of Ovambo warriors, watchful but willing, lining up in their quartiles. Weldon (the man with the crabs) was worried too: “The position now seems to be that Pearson, whom I do not trust as a clear thinker when he writes without symbols, has to be trusted implicitly when he hides in a table of Î-functions, whatever they may be.”
rationale
quite clear, for your formulae, and your justification for applying them?” Galton knew he had not the mathematics to dispute Pearson's formulae; but he fretted that a curve expressed as
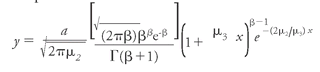
There are two significant points hereâone cultural and one philosophical. The first has to do with the dismal reputation of statistics: that dry and empty sensation in the stomach's core when the subject arises, either in assignment or conversation. Any newspaper reader would be able to make sense of one of Galton's essays, but Pearson carried statistics off into a mathematical thicket that has obscured it ever since. He was a
scientist
and wrote for scientistsâbut this meant that the ultimate tool of science, its measure of certainty, became something the rest of us had to take on trust. Now that the calculation is further entombed, deep within the software that generates statistical analyses, it becomes even more like the pronouncement of an oracle: the adepts may interpret, but we in the congregation can only raise our hands and wonder.
scientist
and wrote for scientistsâbut this meant that the ultimate tool of science, its measure of certainty, became something the rest of us had to take on trust. Now that the calculation is further entombed, deep within the software that generates statistical analyses, it becomes even more like the pronouncement of an oracle: the adepts may interpret, but we in the congregation can only raise our hands and wonder.
The larger point has to do with that certainty. Let us put aside utter skepticism and agree that the stars are
really
thereâor at least something very like them. To admit that our observations of their positions show unavoidable error is no great leap: we are human; we make mistakes. To say these errors fall into a pattern is also an acceptable assumption about reality. Pearson, though, took things further: to his mind, measurements were not attempts at certainty about a thing, but the results of a random process. Observation is like rolling a dieâone with many faces and an unknown shape. The scatter of measurements appears across our chart with as little intrinsic meaning as the record of red and black over two months at Monte Carlo. It is only when we have plotted our distribution curve on that scatter and judged its “goodness of fit” that we can begin to do science: determining the mean value, judging the symmetry of the curve, calculating the standard deviation, looking for outlying values. These
parameters
âa word we toss around with such facilityâare the apparent qualities of a curve that has been fitted to the plot of selected observed measurements of a random variable, in itself an apparent quality of some real thing.
This
is the reality; this is the truth. Whatever is out thereâwhether a new star in Cassiopeia or your child's intelligenceâis only an approximation: an individual manifestation of a random collective process. This view may be right mathematicallyâafter all, the whole point of probability and statistics is to be rigorous about uncertaintyâbut it makes the journey back from rigor to reality a long and hazardous expedition.
really
thereâor at least something very like them. To admit that our observations of their positions show unavoidable error is no great leap: we are human; we make mistakes. To say these errors fall into a pattern is also an acceptable assumption about reality. Pearson, though, took things further: to his mind, measurements were not attempts at certainty about a thing, but the results of a random process. Observation is like rolling a dieâone with many faces and an unknown shape. The scatter of measurements appears across our chart with as little intrinsic meaning as the record of red and black over two months at Monte Carlo. It is only when we have plotted our distribution curve on that scatter and judged its “goodness of fit” that we can begin to do science: determining the mean value, judging the symmetry of the curve, calculating the standard deviation, looking for outlying values. These
parameters
âa word we toss around with such facilityâare the apparent qualities of a curve that has been fitted to the plot of selected observed measurements of a random variable, in itself an apparent quality of some real thing.
This
is the reality; this is the truth. Whatever is out thereâwhether a new star in Cassiopeia or your child's intelligenceâis only an approximation: an individual manifestation of a random collective process. This view may be right mathematicallyâafter all, the whole point of probability and statistics is to be rigorous about uncertaintyâbut it makes the journey back from rigor to reality a long and hazardous expedition.
Florence Nightingale had once approached her great friend Benjamin Jowett, Master of Balliol, with the idea of starting a statistics faculty at Oxford, but nothing came of it. Pearson, thanks to a substantial legacy from Francis Galton, did establish a Department of Eugenics at University College London and the first great statistical journal,
Biometrika
. He fought hard for the recognition of statistics as a separate disciplineâunder, of course, his own banner; practitioners who took too independent a line were usually cast into outer darkness. He brought in armies of assistants to plot curves, judge goodness of fit, and churn out parameters, using new mechanical aids like the Millionaire, a sewing-machine-size calculator that (as its name implied) could handle inputs in seven digits. Many of these assistants were female; indeed, one distinction of statistics is that it served as a secret passage into the fortress of academia for many women who later built distinguished careers. Under Pearson's rule, statistics was no longer just a philosophical vogue, a useful method, a tool for observers and activists, a side branch of mathematicsâit had become a Department with Professors, almost an end in itself. That is how we find it today, in universities, companies, hospitals, and government departments all over the world; and this now seems . . . perfectly normal.
Biometrika
. He fought hard for the recognition of statistics as a separate disciplineâunder, of course, his own banner; practitioners who took too independent a line were usually cast into outer darkness. He brought in armies of assistants to plot curves, judge goodness of fit, and churn out parameters, using new mechanical aids like the Millionaire, a sewing-machine-size calculator that (as its name implied) could handle inputs in seven digits. Many of these assistants were female; indeed, one distinction of statistics is that it served as a secret passage into the fortress of academia for many women who later built distinguished careers. Under Pearson's rule, statistics was no longer just a philosophical vogue, a useful method, a tool for observers and activists, a side branch of mathematicsâit had become a Department with Professors, almost an end in itself. That is how we find it today, in universities, companies, hospitals, and government departments all over the world; and this now seems . . . perfectly normal.
7
Healing
Non est vivere, sed valere vita est. (Life is not living, but living in health)
âMartial,
Epigrams
, VI, lxx
Epigrams
, VI, lxx
Â
Â
Â
Â
Â
Â
W
atching the balls rattle down Galton's quincunx, you feel amazed at order arising out of chaosârandom ricochets settling into the satisfying curve of a normal distributionâbut only if each ball is anonymous. If one ball represents
you,
why should you care that your haphazard path combines with the others to make a neat, coherent picture? You want to know where
you're
going.
atching the balls rattle down Galton's quincunx, you feel amazed at order arising out of chaosârandom ricochets settling into the satisfying curve of a normal distributionâbut only if each ball is anonymous. If one ball represents
you,
why should you care that your haphazard path combines with the others to make a neat, coherent picture? You want to know where
you're
going.
This has always been the dilemma in medicine. In Malraux's
La voie royale,
the hero concludes: “There is no Death . . . there is only I, who am dying.” When the bodyâoldest and closest companionâbegins to give out, the problem is not abstract.
I
hurt,
I
tire,
I
fear: something is wrong with
me;
there must be something you can do for
me.
The doctor's answer may also be couched in those terms, but the science on which it is based has nothing to do with the individual. It is collective and probabilistic. That standby of medical dialogueâ“What chance do I have, Doctor?” “I'd say about sixty percent”âdoes not mean what the patient thinks it means: “You have a sixty percent chance of coming through this crisis” but rather, at best: “I remember a study reporting that, of a thousand people roughly like you, roughly in the same position, four hundred of them died.”
La voie royale,
the hero concludes: “There is no Death . . . there is only I, who am dying.” When the bodyâoldest and closest companionâbegins to give out, the problem is not abstract.
I
hurt,
I
tire,
I
fear: something is wrong with
me;
there must be something you can do for
me.
The doctor's answer may also be couched in those terms, but the science on which it is based has nothing to do with the individual. It is collective and probabilistic. That standby of medical dialogueâ“What chance do I have, Doctor?” “I'd say about sixty percent”âdoes not mean what the patient thinks it means: “You have a sixty percent chance of coming through this crisis” but rather, at best: “I remember a study reporting that, of a thousand people roughly like you, roughly in the same position, four hundred of them died.”
Medicine is a profession long held in honor because it averts fate. Asclepius was considered the son of a god because only someone in touch with the divine could legitimately interfere with illness, previously the province of prayer and sacrifice. Medieval doctors were like priests, drawing knowledge from ancient texts, proceeding by deduction and comparison. As late as the mid-eighteenth century, Galen, Avicenna, and Hippocrates remained the set books in every European medical school: although anatomists had long since shown the heart to be a pump, students still learned that it was some sort of furnace. As of 1750, there were two effective medicines: cinchona bark for malaria and mercury for syphilis; one effective surgical procedure: cutting for bladder stones; and one sensible prescription based on observation: vaccination against smallpox with cowpox. Apart from that (and despite a long tradition of excellent descriptive anatomy going back to Leonardo) all was fluctuating humors, plague-repellent pomanders, purgings, bleedings, clysters, and humbug. Indeed, it is debatable whether going to a doctor at any time before 1880 would have increased or decreased one's chance of survival.
Medicine lacks the key experiments associated with other sciences. Walter Reed's mosquito, Pasteur's rabid boy, and Fleming's gardening boots don't have the clinching perfection of Galileo on his tower. The body has too few ways of letting us know what's wrong, and there are too many potential causes for the same phenomenonâthink how baffled medicine still seems by vague but persistent viral infections. If each symptom pointed to a single cause, we could reason about it deductivelyâa simple procedure, which may explain why the works of the deductive theorists were preserved, in contradiction to observed fact, for so long.
When Quetelet's work first appeared, his new Statistics briefly promised real value in medicine. In 1828 Pierre Charles Alexandre Louis made a quantitative study of the effect of bloodletting on pneumonia and found it essentially useless, thus sparing countless exhausted patients the ordeal of lancet and leech. The vogue for numerical medicine soon died out, however, both because the limited studies hardly merited their broad conclusions, and because of powerful resistance from within the medical profession.
It's tempting to believe that this was simply the reactionary tendency of an elite anxious to hold on to its fees, but there were also more interesting and more sincere objectors. Risueño d'Amador thought that statistics breached the intuitive connection between doctor and patient, the “tact” that allows this unique interaction of person and disease to be understood. Considering disease collectively might actually
reduce
the chance of curing the patient in front of you, since he would be bound to differ from the average. Claude Bernard saw statistics as standing in the way of developing exact knowledge: medicine was simply a young science, which in time would grow into the certainty of physics. Physiologists, he said, “must never make average descriptions of experiments, because the true relations of phenomena disappear in the average.”
reduce
the chance of curing the patient in front of you, since he would be bound to differ from the average. Claude Bernard saw statistics as standing in the way of developing exact knowledge: medicine was simply a young science, which in time would grow into the certainty of physics. Physiologists, he said, “must never make average descriptions of experiments, because the true relations of phenomena disappear in the average.”
The problem arose from having to draw collective conclusions when the way things vary is probably more important than the way they stay the same. Although statistics could reveal broad correlations, as in John Snow's cholera map and Florence Nightingale's bat-wing charts, it had as yet no power to define degrees of action, no method for separating confounded variables, no hope of being scientifically rigorous about the uncertain.
Yet there was an entirely different field of study that tackled these same difficulties: agronomy. In the thirteenth century, when the red-robed
magister
at Oxford's medical schools would explain that all disease was the result of uneven mixture of the hot, wet, cold, and dry elements in the body, a lecturer further down the muddy street, Walter of Henley, was telling students in the estate-management course:
magister
at Oxford's medical schools would explain that all disease was the result of uneven mixture of the hot, wet, cold, and dry elements in the body, a lecturer further down the muddy street, Walter of Henley, was telling students in the estate-management course:
Change yearly your seed corn at Michaelmas, for more increase shall you have of the seed that grew on another man's land than by that which groweth upon your own land. And will you see it? Cause the two lands to be plowed in one day and sow the one with the bought seed and the other with the seed which grew of your own and at harvest you shall find that I say truth.
Other books
Chains (The Club #8) by T. H. Snyder
Sacrificial Magic by Stacia Kane
The Word for World is Forest by Ursula K. Le Guin
Sharing Space (The Complete Series) by Perez, Nina
The Watchers by Ruth Ann Nordin
After The Moon Sets 2 (Werewolf Paranormal Romance) by Taylor, Reba
Betwixt by Melissa Pearl
Crónica de una muerte anunciada by Gabriel García Márquez
The Heart Heist by Kress, Alyssa
Wages of Sin by Kate Benedict