The Particle at the End of the Universe: How the Hunt for the Higgs Boson Leads Us to the Edge of a New World (41 page)
Authors: Sean Carroll

The reason why this fact is so important is that each of the allowed spin measurements represents a new “degree of freedom.” That’s physics-speak for “something that can happen independently of other things happening.” Since what we’re really talking about here are quantum fields, every degree of freedom is a specific way the field can vibrate. For a spin-0 field like the Higgs, there’s only one way it can vibrate. For a spin-1/2 field like the electron, it can have two kinds of vibrations, consisting of clockwise or counterclockwise spinning around whatever axis you choose. (Hard to visualize, admittedly.) A massless spin-1 particle like the photon also has just two kinds of vibrations. But a massive spin-1 particle like the Z boson has three kinds of vibrations: With respect to some axis, it could be spinning clockwise, counterclockwise, or not at all.
This might sound like a confusing mess, but if you go back to the discussion of the Higgs mechanism in Chapter Eleven, it helps make sense of what happens when we spontaneously break a local symmetry. Remember that in the Standard Model, we start (before symmetry breaking) with three massless gauge bosons and four scalar Higgs bosons. Count the number of degrees of freedom: two each for the three massless gauge bosons, one each for the scalars, giving 2 × 3 + 4 = 10. After symmetry breaking, three of the scalars get eaten by the gauge bosons, which become massive, leaving behind one massive scalar that we observe as the physical Higgs boson. Now count the degrees of freedom again: three each for three massive gauge bosons, plus one for the remaining scalar, giving 3 × 3 + 1 = 10. They match. Spontaneous symmetry breaking doesn’t create or destroy degrees of freedom, it just jumbles them up.
Counting degrees of freedom helps explain why gauge bosons are massless without the Higgs. The reason gauge bosons exist in the first place is that there is a local symmetry—one that operates separately at every point in space—and we need to define connection fields that relate the symmetry operations at different points. It turns out that you need precisely two degrees of freedom to define this kind of field. (Trust me here. It’s hard to think of a sensible explanation that doesn’t amount to going through all the math.) When you have a spin-1 or spin-2 particle with just two degrees of freedom, that particle is necessarily massless. The Higgs field is a completely separate degree of freedom; when it gets eaten by the gauge bosons, they now become massive. If there were no extra degrees of freedom lying around, the gauge bosons would have had to remain massless, as they do for the other known forces.
Hopefully this helps explain why physicists were so confident that something like the Higgs must exist, even before it had been discovered. In some sense, it
had
been discovered—three of the four scalar bosons were already there, as the zero-spin parts of the massive W and Z bosons. All we needed to do was find the fourth.
Why fermions are massless without the Higgs
Here is why the fact that fermions have mass is something that demands an explanation in the first place. Notice that the degrees-of-freedom argument we used for the gauge bosons isn’t relevant in this case; a spin-1/2 fermion has two possible spin values whether it’s massive or massless.
Start by thinking of a massive spin-1/2 particle like the electron. Imagine that it’s moving directly away from us, and we measure its spin to be +1/2 along an axis pointing in the direction of its motion. But we can imagine accelerating our own velocity so that we start catching up to the electron—now we’re moving toward it. Nothing intrinsic to the electron has changed, including its spin, but its velocity with respect to us has. We define a quantity called the “helicity” of a particle, which is the spin as measured along the axis defined by its motion. The helicity of the electron goes from being +1/2 to -1/2, and all we did is change our own motion—we didn’t touch the electron at all. Clearly the helicity isn’t an intrinsic feature of the particle; it depends on how we look at it.
Now consider a massless spin-1/2 fermion (like the electron would be without spontaneous symmetry breaking). Let it be moving away from us, and we measure its spin to be +1/2 along an axis defined by its direction of motion, so its helicity is +1/2. In this case, the fermion is necessarily moving at the speed of light (because that’s what massless particles always do). Therefore we can’t catch up to it and change its apparent direction of motion just by accelerating ourselves. Every observer in the universe will observe this massless particle to have a unique value for its helicity. For massless particles, in other words, the helicity is a well-defined quantity no matter who is measuring it, unlike the case for massive particles. A particle with positive helicity is “right-handed” (spinning counterclockwise as it comes toward us), while a negative-helicity particle is “left-handed” (spinning clockwise as it comes toward us).
And the reason why all this matters is because
the weak interactions couple to fermions of one helicity but not the other
. In particular, before the Higgs comes along to break the symmetry, the massless gauge bosons of the weak interactions couple to left-handed fermions and not right-handed ones, and they also couple to right-handed antifermions and not left-handed ones. Don’t ask why that’s the way nature works, except that it’s what we need to fit the data. The strong force, gravity, and electromagnetism all couple equally well to left- and right-handed particles; but the weak force couples to one but not the other. That also explains why the weak interactions violate parity: Looking at the world through a mirror switches right with left.
Having a force couple to one helicity but not the other clearly doesn’t make sense if the helicity is different to observers moving at different speeds. Either the weak force couples to a certain particle, or it doesn’t. If the weak force couples only to left-handed particles and right-handed antiparticles, it must be true that such particles have one helicity or the other once and for all. And that can happen only if they move at the speed of light. Which implies, at last, that they must have zero mass.
If you can swallow that, it helps make sense of some of the dancing around we did while first defining the Standard Model. We said that the known fermions come in pairs, which would be symmetric if it weren’t for the Higgs lurking in empty space. Up and down quarks form a pair, electrons and electron neutrinos form a pair, and so on. But really it’s only the left-handed up and down quarks that form a symmetric pair; there is no local symmetry connecting right-handed up quarks to right-handed down quarks, and likewise for the electron and its neutrino. (In the original version of the Standard Model, neutrinos were thought to be massless, and right-handed neutrinos didn’t even exist. Now we know that neutrinos have a small mass, but the status of right-handed neutrinos remains murky.) Once the Higgs fills space, the weak symmetry is broken, and the observed quarks and charged leptons are all massive, with both right- and left-handed helicities allowed.
Now we see why the Higgs is needed in order for Standard Model fermions to have mass. If the weak interaction symmetry were unbroken, helicity would be a fixed property of each fermion, which means that they all would be massless particles moving at the speed of light. It’s all because the weak interactions can tell left from right. If that weren’t true, there would be no obstacle to fermions simply having mass, with or without the Higgs. Indeed, the Higgs itself is a scalar field with mass, but it’s not as if the Higgs gives mass to itself; it simply has mass, since there’s no reason for it not to.
APPENDIX TWO
STANDARD MODEL PARTICLES
Throughout the book we’ve talked about the various particles of the Standard Model, but not always in a systematic way. Here we provide a summary of the particles and their properties.
There are two types of elementary particles: fermions and bosons. Fermions take up space; that is, you cannot put two identical fermions right on top of each other in precisely the same configuration. They therefore serve as the basis for solid objects, from neutron stars to tables. Bosons can be piled on top of one another as much as you like. They therefore are able to create macroscopic force fields, such as the electromagnetic field and the gravitational field.
The fermions
Let’s consider the fermions first. There are twelve fermions in the Standard Model that fall into strict patterns. Fermions that feel the strong nuclear force are quarks, while those that don’t are leptons. There are six types each of quarks and leptons, arranged into three pairs, each pair forming a generation. It’s a rule that the spin of a fermion must equal an integer plus one half; all the known elementary fermions are spin-1/2 particles.
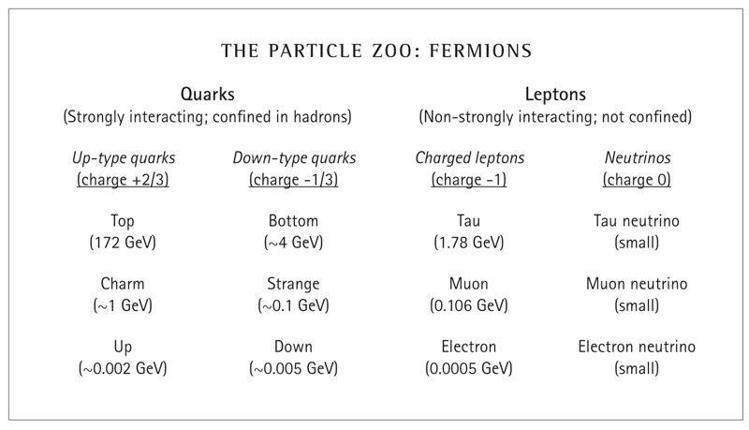
The elementary fermions, with their electric charges and approximate masses. The masses of neutrinos haven’t yet been accurately measured, but they are all lighter than the electron. Quark masses are also approximate; they are hard to measure because quarks are confined inside hadrons.
There are three up-type quarks, with an electrical charge of +2/3 each. In order of increasing mass, they are the up quark, the charm quark, and the top quark. There are also three down-type quarks, with charge -1/3 each: the down quark, the strange quark, and the bottom quark.
Each type of quark comes in three colors. It would be perfectly legitimate to count each color as a separate kind of particle (in which case there would be eighteen types of quarks, not just six), but because the colors are all related by the unbroken symmetry of the strong interactions, we usually don’t bother. All particles with color are confined into colorless combinations known as “hadrons.” There are two simple types of hadrons: mesons, which consist of a quark and an antiquark, and baryons, consisting of three quarks, one each of the three colors red, green, and blue. Protons (two ups and one down) and neutrons (two downs and one up) are both baryons. An example of a meson is the pion, which comes in three types: one with positive charge (up plus antidown), one with negative charge (down plus antiup), and one that is neutral (a mixture of up-antiup and down-antidown).
Unlike quarks, leptons are not confined; each one can move by itself through space. The six leptons also come in three generations, each with one neutral particle and one with charge -1. The charged leptons are the electron, muon, and tau. The neutral leptons are the neutrinos: the electron neutrino, the muon neutrino, and the tau neutrino. Neutrino masses are not well understood and don’t arise in the same way as those of other Standard Model fermions, so we have essentially ignored them in this book. They’re known to be small (less than one electron volt) but not zero.
The twelve different fermions should really be thought of as six different matched pairs of particles. Each charged lepton comes in a pair with its associated neutrino, while the up and down quarks form a pair, as do the charm and strange quarks, and the top and bottom. As an example of this pairing in action, when a W
-
boson decays into an electron and an antineutrino, it’s always an electron antineutrino. Likewise, when a W
-
decays into a muon, it’s always accompanied by a muon antineutrino, and so on. (You would like to say the same thing about the quarks, but they actually mix together in subtle ways.) The particles within each pair would actually have identical properties, if it weren’t for one sneaky influence lurking in the background: the Higgs field. In the world we see, the particles within each pair have different masses and different electrical charges, but that’s only because the Higgs is hiding their underlying symmetric nature.
Is it possible that the quarks and leptons aren’t really elementary, and that they are actually made of an even smaller level of particles? Sure, it’s possible. Physicists don’t have a vested interest in the current particles being truly elementary; they would love to find yet more mysteries hidden within them, and they have spent a great deal of time inventing models along those lines and testing them experimentally. The hypothetical particles that could make up quarks and leptons even have a name: “preons.” What they don’t have is any experimental evidence, or, for that matter, any compelling theory. The consensus these days is that quarks and leptons seem to be truly elementary, rather than being composites of some other kind of particle, but we can always be surprised.
The bosons
Now we turn to the bosons, which always have integer spins. The Standard Model includes four types of gauge bosons, each arising from local symmetries of nature, and corresponding to a certain force.
Photons, which carry the electromagnetic force, are massless, neutral, spin-1 particles. Gluons, which carry the strong nuclear force, are also massless, neutral, and spin-1. A major difference is that gluons do carry color, so they are confined inside hadrons just like quarks are. Because of these colors there are actually eight different kinds of gluons, but once again they are related by an unbroken symmetry, so we don’t even bother to give them specific labels.