Three Roads to Quantum Gravity (23 page)

The search for evidence of supersymmetry is a major priority of experiments now under way at particle accelerators. String theorists very much hope that evidence for supersymmetry will be found. If supersymmetry is not found experimentally, it would still be possible to concoct a string theory that agrees with experiment, but this would be a less happy outcome than if experimental support for supersymmetry were forthcoming.

There is obviously something very wonderful about string theory. Among its strong points are the natural way it leads to a unification of all particles and forces, and the fact that there are many consistent string theories that include gravity. String theory is also the perfect realization of the hypothesis
of duality discussed in Chapter 9. Also, it cannot be overemphasized that in the language in which it is understood - that of diagrams corresponding to quantum particles moving against a background spacetime - string theory is the only known way of consistently unifying gravity with quantum theory and the other forces of nature.

What is very frustrating is that in spite of this, string theory does not seem to fully incorporate the basic lesson of general relativity, which is that space and time are dynamical rather than fixed, and relational rather than absolute. In string theory, as it has so far been formulated, the strings move against a background spacetime which is absolute and fixed. The geometry of space and time is usually presumed to be fixed for ever; all that happens is that some strings move against this fixed background and interact with one another. But this is wrong, because it replicates the basic mistake of Newtonian physics in treating space and time as a fixed and unchanging background against which things move and interact. As I have already emphasized, the right thing to do is to treat the whole system of relationships that make up space and time as a single dynamical entity, without fixing any of it. This is how general relativity and loop quantum gravity work.

Still, science is not made from absolutes. The progress of science is based on what is possible, which means that it often makes sense to do what is practical, even if it seems to go against established principles. For this reason, even if it is ultimately wrong, it may still be useful to follow the background dependent approach as far as it will go, to see whether there is a consistent picture in which we can answer questions such as what happens when two gravitons moving in empty spacetime scatter from each other. As long as we remember that such a picture can give at best an approximate description this can be an important and necessary step in the discovery of the quantum theory of gravity.

Another main shortcoming of string theory is that is not one theory, but a whole class of theories, so it does not lead to many predictions about the elementary particles. This shortcoming is closely related to the problem of background
dependence. Each string theory moves against a different background spacetime, so to define a string theory one must first fix the dimension of space and the geometry of spacetime. In many cases space has more dimensions than the three we observe. This is explained by the hypothesis that in our universe the extra dimensions are curled up too tightly for us to perceive directly. We say that the extra six dimensions have been compactified. Since string theory is simplest if the world has nine spatial dimensions, this leads to a picture in which many of the different consistent string theories in three dimensions can be understood as arising from different ways of choosing the structure of a hidden six-dimensional space.

There are at least hundreds of thousands of ways in which the six extra dimensions may be compactified. Each way corresponds to a different geometry and topology for the extra six dimensions. As a result there are at least that many different string theories that are consistent with the basic observation that the world has three large spatial dimensions. Furthermore, each of these theories has a set of parameters that describe the size and other geometric properties of the six compactified dimensions. These turn out to influence the physics that we see in the three-dimensional world. For example, the geometry of the extra dimensions influences the masses and the strengths of the interactions of the elementary particles we observe.

It is most likely irrelevant whether these extra dimensions exist in any literal sense. If one is drawn to a picture of our three-dimensional ‘reality’ embedded in some higher-dimensional realm, then one can believe in the extra dimensions, at least as long as one is working in this background dependent picture. But these extra dimensions can also be seen as purely theoretical devices which are useful for understanding the list of consistent string theories in three dimensions. As long as we stay on the background dependent level, it does not really matter.

As a result, although it is a unified theory, string theory in its present form makes few predictions about the physics we actually observe. Many different scenarios for what the new, more powerful particle accelerators will find are consistent
with one version of string theory or another. Thus, not only does string theory lack experimental confirmation, but it is hard to imagine an experiment that could be done in the next several decades that could definitively confirm or reject it. Nor is there anything special, from the point of view of string theory, about having six out of nine dimensions compactified while the other three are left large. String theory can easily describe a world in which any number of dimensions, from nine down to none at all, are left large.

String theory thus indicates that the world we see provides only a sparse and narrow sampling of all possible physical phenomena, for if true it tells us that most of the dimensions and most of the symmetry of the world are hidden. Still, many people do believe in it. This is partly because, however incomplete its present formulation may be, string theory remains the one approach that unifies gravity with the other forces consistently at a background dependent level.

The main problem in string theory, then, is how to see beyond it to a theory which will incorporate the successes of string theory while avoiding its weaknesses. One approach to this problem begins with the following question. What if there were a single theory that unified the different string theories by interpreting each of its solutions as one of the consistent string theories? The different string theories, together with the spacetimes they live in, will not be put in as absolutes. Rather they will all arise from solutions of this new theory. Note that the new theory could not be formulated in terms of any objects moving against a fixed spacetime background, because its solutions would include all the possible background spacetimes. The different solutions of this fundamental theory would be analogous to the different spacetimes which are all solutions to the equations of general relativity.

Now we can argue by analogy in the following way. Let us take any spacetime which is a solution to the Einstein equations, and wiggle some matter within it. This will generate gravitational waves. These waves move on the original spacetime like ripples moving on the surface of a pond. We can make ripples in the solution of our fundamental theory in the same way. What if these gave rise not to waves
moving on the background, but to strings? This may be hard to visualize, but remember that according to the hypothesis of duality strings are just a different way of looking at a field, like the electric field. And if we wiggle a field we get waves. The wiggles in the electric and magnetic field are after all nothing but light. But if duality is true, there must be a way to understand this in terms of the motion of strings through space.

If this picture is correct, then each string theory is not really a theory in its own right. It is no more than an approximate description of how ripples may move against a background spacetime which itself is a solution to another theory. That theory would be some extension of general relativity, formulated in terms that were relational and background independent.

This hypothesis would, if true, explain why there are so many different string theories. The solutions to the fundamental theory will define a large number of different possible universes, each described in terms of a different space and time.

It remains only to construct this single, unifying string theory. This is a project that a few people are working hard on, and I must confess it is something I also am spending a lot of time on. There is presently no agreed upon form of this theory, but at least we have a name for it - we call it M theory. No one knows what the M stands for, which we feel is appropriate for a theory whose existence has so far only been conjectured.

These days, string theorists spend much of their time looking for evidence that M theory exists. One strategy which has been very successful is to look for relationships between different string theories. A number of cases have been found in which two apparently different versions of string theory turn out to describe exactly the same physical phenomena. (In some cases this is seen directly; in others the coincidence is apparent only certain approximations or from studying simplified versions of the theories in which extra symmetries have been imposed.) These relationships suggest that the different string theories are part of a larger theory. The
information about these relationships can be used to learn something about the structure M theory must have, if it exists. For example, it gives us some information about the symmetries that M theory will have. These are symmetries that extend the idea of duality in a major way, which could not be done within any single string theory.

Another very important question is whether M theory describes a universe in which space and time are continuous or discrete. At first it seems that string theory points to a continuous world, because it is based on a picture of strings moving continuously through space and time. But this turns out to be misleading, for when looked at closely string theory seems to be describing a world in which space has a discrete structure.

One way to see the discreteness is to study strings on a space that has been wrapped up, so that one dimension forms a circle (
Figure 37
). The circle which has been wrapped up has radius R. You might think that the theory would get into trouble if we allowed R to get smaller and smaller. But string theory turns out to have the amazing property that what happens when R becomes very small is indistinguishable from what happens when R becomes very large. The result is that there is a smallest possible value for R. If string theory is right, then the universe cannot be smaller than this.

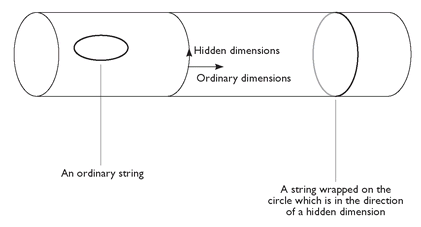
A cylinder is a two-dimensional space in which one direction is a circle. We see a string wrapped on the circle. This is typical of ideas of how the extra dimensions are hidden; the horizontal direction is typical of the three ordinary directions, while the vertical direction stands for one of the hidden dimensions. Time is not indicated here.

There is a pretty simple explanation for this, which I hope will at least give you a taste of the kind of reasoning that permeates the study of string theory. The reason why R has a smallest possible value has to do with the fact that there are two different things a string can do when wrapped around a cylinder (it is said to have two degrees of freedom). First, it can vibrate, like a guitar string. Since the radius of the cylinder is fixed there will be a discrete series of modes in which the string can vibrate. But the string has another degree of freedom, because one can vary the number of times it is wrapped around the cylinder. Thus there are two numbers that characterize a string wrapped around a cylinder: the mode number and the number of times it is wrapped.

It turns out that if one tries to decrease the radius of the cylinder, R, below a certain critical value, these two numbers just trade places. A string in the 3rd mode of vibration wrapped 5 times around a cylinder with R slightly smaller than the critical value becomes indistinguishable from a string wrapped 3 times around a cylinder slightly larger than the critical value, when it is in the 5th mode of vibration. The effect is that every mode of vibration of a string on a small cylinder is indistinguishable from a different mode of a string wrapped on a large cylinder. Since we cannot tell them apart, the modes of strings wrapped around small cylinders are redundant. All the states of the theory can be described in terms of cylinders larger than the critical value.

Another way to see the discreteness is to imagine a string going by at very nearly the speed of light. It would appear to contain a set of discrete elements, each of which carries a certain fixed amount of momentum. These are called string bits, and they are shown in
Figure 38
. The more momentum a string has, the longer it is, so there is a limit to the size of an object that can be resolved by looking at it with a string. But since, according to string theory, all the particles in nature are actually made up of strings, then, if the theory is right, there is
a smallest size. Just as there is a smallest piece of silver, which is a silver atom, there is a smallest possible process that can propagate, and that is a string bit.